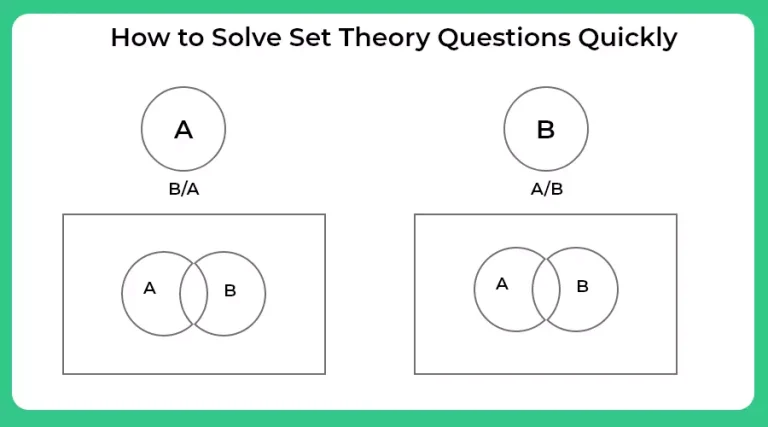
How To Solve Set Theory Questions Quickly

August 10, 2023

Solving Set Theory Quickly and Easily

Definition for Set Theory – Set Theory is a branch of Mathematics that deals with the properties of well- defined collections of an object. In other words, its natural habit for all of us to classify similar things into groups. These groups are known as Set. This page is basically to help students and help them with a query of – “How To Solve Set Theory Questions Quickly.”

We are going to provide you the best methods to solve the questions quickly. The best way to solve the question is by practicing them and before practicing we need to see the Solved Examples. Here are a few solved examples which will definitely help your query of – “How To Solve Set Theory Questions Quickly.”.

How to solve Perimeter, Area and Shapes
How To Solve Set Theory

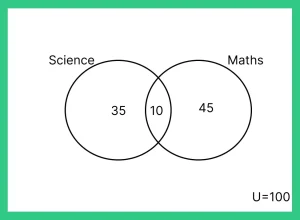
Question 1 – In a class of 100 students, 35 like science and 45 like math. 10 like both. How many students like neither of them?

OPTIONS
a) 30

b) 24

c)31

d)18

Explanations – Total number of students, n(µ) = 100
Number of science students, n(S) = 35
Number of math students, n(M) = 45
Number of students who like both, n(M∩S) = 10
Number of students who like either of them,
n(MᴜS) = n(M) + n(S) – n(M∩S)
=> 45+35-10 = 70

Number of students who like neither = n(µ) – n(MᴜS) = 100 – 70 = 30

CORRECT ANSWER(A)

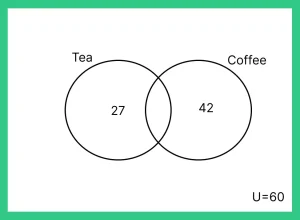
set theory tips

Question 2 – In a group of 60 people, 27 like tea and 42 like coffee and each person likes at least one of the two drinks. How many like both coffee and tea?

OPTIONS
a) 12
b) 3
c) 8
d) 9
 
Explanation
 Let Tea= Set of people who like tea
Coffee = Set of people who like coffee
Given,
(T∪C) = 60
 n(T) = 27
 n(C) = 42
 n(T) + n(C) – n(T ∪ C)
= 27 + 42 – 60
= 69 – 60 = 9
= 9

Therefore, 9 people like both tea and coffee.

CORRECT ANSWER (D)

set theory

 

Question 3 – Let A and B be two finite sets such that n(A) = 24, n(B) = 37 and n(A ∪ B) = 46, find n(A ∩ B).

OPTIONS

a)12

b) 15

c) 8

d) 4

Explanation 

Using the formula n(A ∪ B) = n(A) + n(B) – n(A ∩ B).

then n(A ∩ B) = n(A) + n(B) – n(A ∪ B)
= 24 +37 – 46

= 61- 46
= 15

CORRECT ANSWER (B)

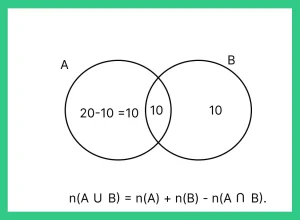
set theory tips

Question 4 – A class consisted of 50 students, 20 were studious and liked to do physical activities also and 10 only liked to study how many students came under none of the above category?

OPTIONS

a)12

b) 25

c) 30

d) 4

Explanation 

 Using the formula n(A ∪ B) = n(A) + n(B) – n(A ∩ B).

then n(A ∩ B) = n(A) + n(B) – n(A ∪ B)
= 20+10 – 10-50

= 30

CORRECT ANSWER (C)

set theory tips

Question 5 – In a college 500 students speak Hindi 200 speak English and 200 speak both Hindi and English, find the number of students in  the college?

OPTIONS

a)300

b) 200

c) 450

d) 500

 Explanation

Number of students who speak Hindi =n(H)=500

Number of students who speak English =n(E)=200

Number of students who speak both =n(HUE)=200

U=n(H)+n(E)-n(HUE)= 500+200-200=

CORRRECT ANSWER(D)

set theory

Prime Course Trailer

Related Banners

Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription

Get Prime

Also Check Out

Set Theory Question and Answers
Important Formulas Related To Set Theory
Set Theory Important Tips ,Tricks and Shortcuts
Branding