Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
Arithmetic, Geometric And Harmonic Progressions Formulas
Basic Formulas for AP, GP and HP with definition
Basic Formulas for AP, GP and HP with definition
In this Page you will Find Formulas for AP and GP and HP as well as definition also. These are Standard Formulas to Solve any Types of Problems of AP and GP and HP.
AP stands for Arithmetic progression
A series of number is termed to be in Arithmetic progression when the difference between two consecutive numbers remain the same.This constant difference is called the common difference.
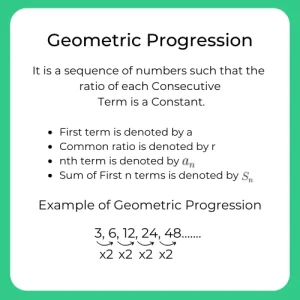
GP stands for Geometric progression
A geometric progression is a sequence of numbers in which each term is obtained by multiplying the previous term by a constant ratio. This constant ratio is called the common ratio.
HP stands for Harmonic progression
A harmonic progression is a sequence of numbers in which each term is the reciprocal of an arithmetic progression.

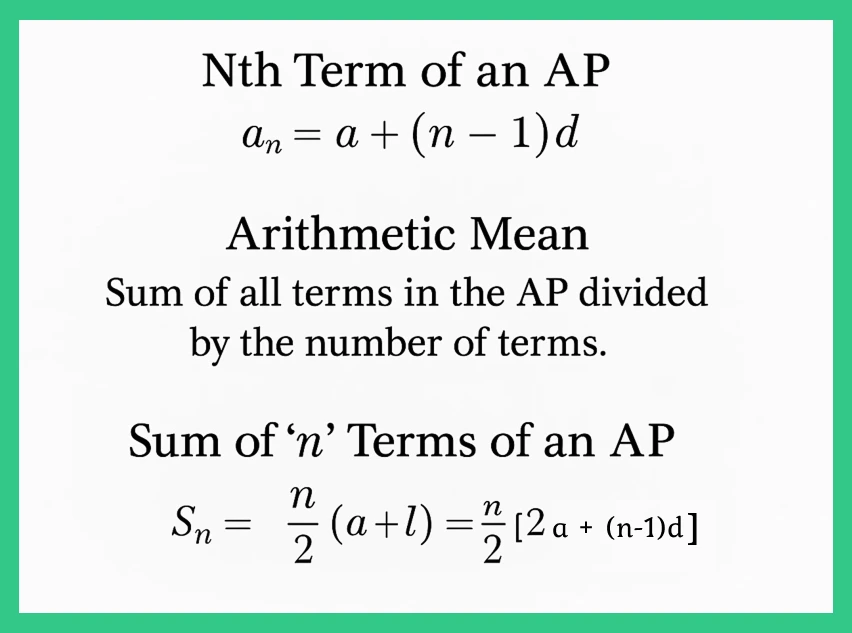
Formulas of Arithmetic Progression (A.P)
In A.P. the next number can be obtained by adding or subtracting the constant number to the previous in the sequence. Therefore, this constant number is known as the common difference(d).
Suppose, if ‘a’ is the first term and ‘d’ be the common difference, then
- nth term of an AP: (a + (n-1)d)
- Arithmetic Mean: Sum of all terms in the AP divided by the number of terms in the AP.
- Sum of ‘n’ terms of an AP: (0.5n*(first term + last term) = 0.5n*[2a + (n-1)d])

Formulas of Geometric Progression (G.P)
Suppose, if ‘a’ is the first term and ‘r’ be the common ratio, then
- Formula for nth term of GP = a r n-1
- Geometric mean = nth root of the product of ‘n’ terms in the GP.
- Formula to find the geometric mean between two quantities a and b = [latex]\sqrt{ab}[/latex]
- Formula to find the sum of the number of terms in a GP
Let ‘a’ be the first term, ‘r’ be the common ratio and ‘n’ be the number of terms
- if r>1 , then , [latex]s_{n} = a \times \frac{r^{n} -1}{r-1}[/latex]
- if r<1 , then , [latex]s_{n} = a \times \frac{1-r^{n}}{1-r}[/latex]
Sum of infinite terms in a GP(r<1) [latex]\frac{a}{1-r}[/latex]

Definition of Harmonic Progression (H.P)
Harmonic progression is the series when the reciprocal of the terms are in AP.
For example, [latex]\frac{1}{a}, \frac{1}{ (a + d)}, \frac{1}{(a + 2d)}[/latex]…… are termed as a harmonic progression as a, a + d, a + 2d are in Arithmetic progression.
- First term of a HP is [latex]\frac{1}{a}[/latex]
- There are many Application of Harmonic Progressions.
Formulas of Harmonic Progression (H.P)
- The nth term in HP is identified by, [latex]{a_{n}} = \frac{1}{a+(n-1)d}[/latex]
- To solve any problem in harmonic progression, a series of AP should be formed first, and then the problem can be solved.
- For two terms ‘a’ and ‘b’,
Harmonic Mean = [latex]\frac{(2ab)}{ (a + b)}[/latex]


Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Relationship Between Arithmetic Mean, Harmonic Mean, and Geometric Mean of Two Numbers
If GM, AM and HM are the Geometric Mean, Arithmetic Mean and Harmonic Mean of two positive numbers respectively, then
GM2 = AM x HM
Using Formulas of A.P, G.P and H.P in Questions
Question 1: The sum of the first 5 terms of a geometric progression is 124. If the common ratio is 2, what is the first term of the progression?
a) 2
b) 4
c) 8
d) 16
Solution:
The sum of the first n terms of a geometric progression is given by S =[latex]\frac{ a (r^{n}-1)}{r-1}[/latex] , where S is the sum, a is the first term, r is the common ratio, and n is the number of terms.
Given S = 124, r = 2, and n = 5, we can substitute these values into the formula and solve for a:
124 = [latex]\frac{ a (2^{5}-1)}{(2-1)}[/latex]
124 = [latex]\frac{ a (32-1)}{1}[/latex]
124 = a(31)
= [latex]\frac{ 124}{31}[/latex] = 4
Therefore, the first term of the progression is 4.
Correct answer: b
Question 2: The 5th term of an arithmetic progression is 17 and the 10th term is 32. What is the common difference?
a) 3
b) 4
c) 5
d) 6
Solution: The nth term of an arithmetic progression is given by a + (n-1)d, where a is the first term, d is the common difference, and n is the term number. Given the 5th term = 17 and the 10th term = 32, we can set up two equations:17 = a + (5-1)d
32 = a + (10-1)d
Solving these equations simultaneously, we get:17 = a + 4d
32 = a + 9d
Subtracting the first equation from the second equation, we get:
32 – 17 = (a + 9d) – (a + 4d) 15 = 5d
d = 3
Therefore, the common difference is 3.
Correct option :a
Question 3: Find t10 and S10 for the following series: 2, 9,16
a) 65,535
b) 55,330
c) 45,440
d) 35,445
Solution:
To find the 10th term (t10) and the sum of the first 10 terms (S10) for the given series, we need to determine the pattern of the series.
Looking at the series, we can observe that each term is obtained by adding 7 to the previous term.
Therefore, the common difference (d) is 7.
To find t10, we can use the formula for the nth term of an arithmetic progression
(AP): tn = a + (n-1)d
Here, a is the first term, which is 2, n is the term number, which is 10, and d is the common difference, which is 7.
t10 = 2 + (10-1) * 7
t10 = 2 + 9 * 7
t10 = 65
So, the 10th term (t10) of the series is 65.
To find S10, we can use the formula for the sum of the first n terms of an arithmetic progression:
Sn = (n/2) * (2a + (n-1)d)
Here, a is the first term, which is 2, n is the number of terms, which is 10, and d is the common difference, which is 7.
S10 = (10/2) * (2 * 2 + (10-1) * 7)
S10 = 5 * (4 + 9 * 7)
S10 = 5 * (4 + 63)
S10 = 5 * 67 S10 = 335
So, the sum of the first 10 terms (S10) of the series is 335.
Correct answer : a
Question 4: A number 39 is divided into three parts which are in A.P. and the sum of their squares is 515. Find the largest number.
(a) 17
(b) 15
(c) 13
(d) 11
Solution:
Let the three numbers be (a-d), a, and (a+d), where a is the middle term and d is the common difference.
According to the given information, we have:
(a-d) + a + (a+d) = 39 (Sum of the three numbers is 39)
((a-d)^2) + (a^2) + ((a+d)^2) = 515 (Sum of their squares is 515)
Expanding the second equation, we get:
a^2 – 2ad + d^2 + a^2 + a^2 + 2ad + d^2 = 515
3a^2 + 2d^2 = 515
Simplifying the equation, we have:
3a^2 + 2d^2 = 515 —-(1)
From the first equation, we have:
3a = 39
a = 13
Substituting the value of a in equation (1), we get: 3(13^2) + 2d^2 = 515
507 + 2d^2 = 515
2d^2 = 8 d^2 = 4
d = 2
Now, we can find the three numbers:
(a-d) = 13-2 = 11
a = 13
(a+d) = 13+2 = 15
Therefore, the largest number is 15.
Correct answer: 15.
Question 5: The sum of the first and the third term of a geometric progression is 15 and the sum of its first three terms is 21. Find the progression.
(a) 3,6,12…
(b) 12, 6, 3…
(c) Both of these
(d) None of these
Solution:
Let’s assume the first term of the geometric progression is “a” and the common ratio is “r”.
According to the given information, the sum of the first and third terms is 15, which can be expressed as:
a + ar^2 = 15 —-(1)
The sum of the first three terms is 21, which can be expressed as:
a + ar + ar^2 = 21 —-(2)
To solve these equations, we can subtract equation (1) from equation (2) to eliminate “a”:
(a + ar + ar^2) – (a + ar^2) = 21 – 15
ar = 6
Now, we can substitute this value of “ar” into equation (1):
a + (6/r) = 15
Multiplying both sides by “r” to eliminate the fraction:
ar + 6 = 15r
Rearranging the equation:
15r – ar = 6
r(15 – a) = 6
Since “r” cannot be zero, we can divide both sides by (15 – a):
r = 6 / (15 – a)
Now, let’s analyze the answer choices:
(a) 3, 6, 12…
If we substitute “a = 3” into the equation for “r”, we get:
r = 6 / (15 – 3) = 6/12 = 1/2
However, this value of “r” does not satisfy the given conditions, as the sum of the first and third terms is not 15.
(b) 12, 6, 3…
If we substitute “a = 12” into the equation for “r”, we get:
r = 6 / (15 – 12) = 6/3 = 2
This value of “r” satisfies the given conditions, as the sum of the first and third terms is indeed 15.
Therefore, the correct answer is 12, 6, 3…
Correct answer: b
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Arithmetic Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Geometric Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Harmonic Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Arithmetic Progressions –
Questions
Formulas
How to Solve Quickly
Tricks & Shortcuts - Geometric Progressions –
Questions
Formulas
How to Solve Quickly
Tricks & Shortcuts - Harmonic Progressions –
Questions
Formulas
How to Solve Quickly
Tricks & Shortcuts

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




awesome
Thank you for your appreciation and also we want you to know that we are more than happy to help you and serve you better!!!
aawsome
awesome