Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
How To Solve Arthmetic, Geometric and Harmonic Progression Quickly
Solve AP, GP and HP Questions Quickly
In this Page you will learn How to Solve AP and GP and HP Questions Quickly through different types of Questions. This topic is very important from examination point of view. That’s why this will helps in different examinations.
Quick methods to solve AP, GP and HP
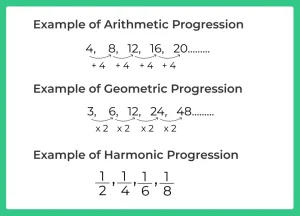
How to solve AP, GP and HP Questions quickly
Arithmetic Progression (AP)
- Identify the first term (a) and the common difference (d) of the sequence.
- Use the formula for the nth term of an AP: a + (n-1)d.
- Use the formula for the sum of the first n terms of an AP: n/2[2a + (n-1)d].
Geometric Progression (GP)
- Identify the first term (a) and the common ratio (r) of the sequence.
- Use the formula for the nth term of a GP: ar^(n-1).
- Use the formula for the sum of the first n terms of a GP: a(1-r^n)/(1-r).
Harmonic Progression (HP)
- Identify the first term (a) and the common difference (d) of the sequence.
- Use the formula for the nth term of an HP: 1/(a+(n-1)d).
- Use the formula for the sum of the first n terms of an HP: n/[2a+(n-1)d].
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Type 1: AP questions
Question 1.
Find the first term of the AP series in which 10th term is 6 and 18th term is 70.
Options:
- 76
- – 76
- 66
- – 66
Solution:
10th term = (a + 9d) = 6….(1)
18th term = (a + 17d) = 70 ……. (2)
On solving equation 1 and 2
We get, d = 8
Put the value of d in equation 1
(a + 9d) = 6
a + 9 x 8 = 6
a + 72 = 6
a = -66
Correct option: 4
Question 2.
Find the nth term of the series 3, 8, 13, 18,…,
Options:
- 2(2n+ 1)
- 5n + 2
- 5n – 2
- 2(2n – 1)
Solution:
The given series is in the form of AP.
first term a = 3
common difference d = 5
We know that, nth term = tn = a + (n-1)d
Therefore, tn = 3 + (n-1) 5
= 3 + 5n – 5
= 5n – 2
Correct option: 3
Question 3.
The series 28, 25,……. -29 has 20 terms. Find out the sum of all 20 terms?
Options:
- -10
- -12
- 10
- 12
Solution:
a =28, d= -3 (25 – 28), l = -29, n = 20
Sum of all n-terms = Sn = \frac{n}{2}(a+l)
S20 = \frac{20}{2} (28 + (-29))
S20 = -10
Correct option: 1
Type 2: GP questions
Question 1.
Find the sum of the following infinite G. P. \frac{1}{3}, \frac{1}{9}, \frac{1}{27}, \frac{1}{81}…….
Options:
- \frac{1}{3}
- \frac{2}{3}
- \frac{1}{5}
- \frac{1}{2}
Solution:
a = \frac{1}{3}, r = \frac{\frac{1}{9}}{\frac{1}{3}} = \frac{1}{3}
Required sum = \frac{a}{(1-r)}
= \frac{\frac{1}{3}}{(1-\frac{1}{3})}
= \frac{\frac{1}{3}}{\frac{2}{3}}
= \frac{1}{2}
Correct option: 4
Question 2.
Find the G. M. between \frac{4}{25} and \frac{196}{125}
Options:
- \frac{28}{5}
- \frac{28}{25}
- \frac{8}{25}
- \frac{14}{5}
Solution:
Geometric mean \sqrt{ab}
GM = \sqrt{\frac{4}{25}\times \frac{196}{25}}
GM = \frac{28}{25}
Correct option: 2
Question 3.
Find the number of terms in the series 1, 3, 9 , ….19683
Options:
- 10
- 8
- 6
- 7
Solution:
In the given series,
a1 = 1, r = \frac{3}{1} = 3, an =19683
=> 19683 = 1 x (3n-1)
=>19683 = 3n-1
=>39 = 3n-1
=>9 = n-1
n = 10
Correct option: 1
Type 3: HP questions
Question 1:
If the 6th term of H.P. is 10 and the 11th term is 18. Find the 16th term.
Options:
- 90
- 110
- 85
- 100
Solution:
6th term = a + 5d = \frac{1}{10}……(1)
11th term = a + 10d = \frac{1}{18}……(2)
On solving equation 1 and 2 we get, d = \frac{-2}{225}
Put value of d in equation 1
a + 5d = \frac{1}{10}
a + 5 \times \frac{-2}{225} = \frac{1}{10}
a = \frac{13}{90}
Now, 16th term = a + 15d = \frac{13}{90} + 15 \times \frac{- 2}{225}
= \frac{13}{90} – \frac{30}{225}
= \frac{1}{90}
Therefore 16th term = 90
Correct option: 1
Question 2.
Find the Harmonic mean of 6, 12, 18
Options:
- 10.12
- 9.62
- 9.81
- 8.10
Solution:
We know that,
HM = \frac{n}{s}
where , s = (\frac{1}{a})+(\frac{1}{b})+(\frac{1}{c})
s=(\frac{1}{6})+(\frac{1}{12})+(\frac{1}{18})
=(\frac{11}{36})
HM = \frac{n}{s}=\frac{3}{(\frac{11}{36})}
HM = 3\times \frac{36}{11}
HM = \frac{108}{11}
HM=9.81
Correct option: 3
Question 3.
What is the relation between AM, GM, and HM?
Options:
- AM x HM = GM2
- AM / HM = GM
- AM + HM = GM2
- AM – HM = GM2
Solution:
AM = \frac{a+b}{2}
GM = \sqrt{ab}
HM = \frac{2ab}{a+b}
Therefore AM x HM = GM2
\frac{a+b}{2} \times \frac{2ab}{a+b}= ab
Correct option: 1
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Arithmetic Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Geometric Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Harmonic Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Arithmetic Progressions –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Geometric Progressions –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Harmonic Progressions –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts
Login/Signup to comment

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Let P = {2, 3, 4, ………. 100} and Q = {101, 102, 103, ….. 200}. How many elements of Q are there such that they do not have any element of P as a factor ?
what is the method to find the prime no. between the numbers as soon as possible ?
Kindly drop your query on 9711936107, then our mentor will resolve all your queries
type 3 harmonic progression. Please explain
Question 2.
Find the Harmonic mean of 6, 12, 18
Options:
10.12
10.9
10.06
6.10
Solution:
We know that,
HM = 3/0.298
HM = 10.06
Correct option: C
formula= n/(1/a1 +1/a2 + 1/a3)
6,12,18 we have 3 numbers
so n=3
then 3/(1/6 + 1/12 +1/18)
ormula= n/(1/a1 +1/a2 + 1/a3)
6,12,18 we have 3 numbers
so n=3
then 3/(1/6 + 1/12 +1/18)
after using this formula we get 9.868 answer
Find the Harmonic mean of 6, 12, 18
Options:
10.12
10.9
10.06
6.10
Solution:
We know that,
HM = 3/0.298
HM = 10.06 ——-???
Please explain
type 3 harmonic progression.
Question 2.
Find the Harmonic mean of 6, 12, 18 Options: 10.12
10.9
10.06
6.10
Solution:
We know that, HM = 3/0.298 HM = 10.06 Correct option: C
These are very useful and easy. Tq prepinsta