Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
How To Solve Averages Questions Quickly
How To Solve Averages Questions Quickly
An Averages is the concept for the Aptitude and other competitive exams. Most of us have a fair understanding of the average or mean but we have not knowledge regarding How to Solve Problems on Averages Quickly. However, the questions from Average which appear in competitive exams are tricky and often calculation intensive if we apply the standard approach.

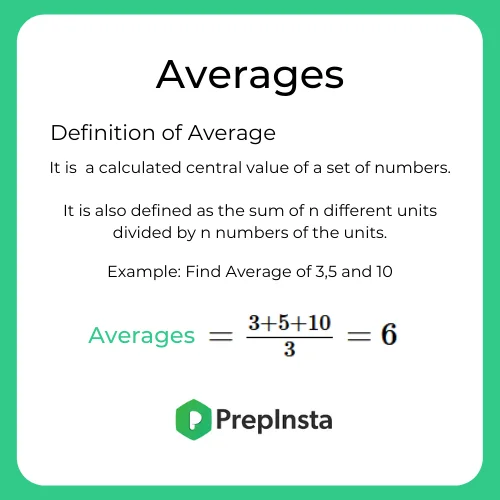
How To Solve Problems On Averages:-
Mathematically, it is defined as the ratio of summation of all the data to the number of units present in the list.
Average = \mathbf{\frac{X_{1}+X_{2}+X_{3}+X_{4}+…..X_{n}}{n}}
OR
Average = \frac{\text{Sum of Observations}}{\text{Total Number of Observations}}
How to Solve Average Speed and Velocity Problems
- Average Speed : Average speed is calculated using the below formula
Average Speed = \mathbf{\frac{\text{Total Distance}}{\text{Total Time}}}
Also , Formula to calculate Average speed when X travels at speed ‘a’ and ‘b’ for the same amount of time is \mathbf{\frac{a+b}{2}}
- Average Velocity : We calculate Average Velocity using the below formula : \mathbf{\frac{Displacement}{Total Time}}
Type 1: How to Solve Problems on Averages Weights and Ages
Question 1 : The average age of 39 boys and a girl is are 11 years. If the age of the girl is excluded the average age of the group is reduced by 1. What is the age of the girl ?
Solution : The average of 39 boys + 1 girl = 11
Sum of ages of 39 boys + 1 girl = 11x (39+1)= 440 ……………… Eqn (i)
When the girl is excluded from the average age the new average = 11- 1= 10
The sum of the ages of 39 boys= 39x 10= 390 ………………Eqn(ii)
Girl’s age= (i)- (ii)= 440- 390= 50
Alternatively,
Alternatively : When the girl leaves the group, she takes with her 1 year (i.e the change in the new average) from each of the 39 students along with the 11 years of her average age.
So the age of the girl will (39 x 1)+ 11 that is 50 years.
Question 2 : The average weight of 10 a group of persons increases by 10 kg when a new person replaces one of the persons from the group who weighs 60kg. Calculate the weight of the new person.
Solution : Total increase in weight = 10 x 10 = 100 kg
Weight of new person = 60+100 =160 kg
Question 3 : The age of Yogesh at the time of his wedding was 27 years, while the age of his wife at that time was 25 years. Four years after their marriage the average age of Yogesh, his wife, and his son is 21 years. Find Age of Yogesh’s son.
Solution : Let the current age son be x
Ages of Yogesh and his wife after 4 years of their marriage = 27+4 = 31 and 25+4 =29 years respectively.
Average age of the family =21
=>\frac{(31+29+x)}{3} = 21
x + 60 = 63
x = 63-60
x = 3 year’s
Therefore, the current age Yogesh’s son is 3 years.
Type 2: How to Solve Problems on Average marks and scores
Question 1 : The batting average of Sachin in 15 innings is 55. The difference between the runs of his best and worst innings is 65. Excluding the best and the worst innings the average of 13 innings played by Sachin is 50. Calculate Sachin’s best score.
Solution : Total score of Sachin in 15 innings = 55 x 15 = 825
Total score of Sachin in 13 innings (excluding his best and worst inning’s scores) = 13 x 50 = 650
Sum of Sachin’s score in his best and worst innings = 825-650 = 175
The difference of Sachin’s score in his best and worst innings = 65
B+W = 175…(1)
B-W = 65…(2)
adding equation 1 and 2; (1)+(2)
2B = 240
B = 120
Therefore, Sachin’s score in his best innings was 120 runs.
Question 2 : The average marks of a class gets increased by 5 when Shikha’s marks are wrongly entered as 75 instead of 55. Find the number of students in the class.
Solution : Let the total strength of class be n.
Let sum of scores of rest of students is x
Let the average when marks of Shikha are 75 = A1= \frac{75+x}{n}
The average of class when shikha marks is 55 = A2 = \frac{(57+x)}{n}
A1-A2 = 5
\frac{(75+x)}{n} – \frac{(55+x)}{n} =520 = 5n
n = 4
Therefore, the total number of students in the class is 4.
Question 3 : A team of 5 players participated in a competition. The best player of the team scored 50 points. Had he scored 80 points, the average score of the team would have been 75. Calculate the total points scored by the team.
Solution : Let the sum of of points scored by 4 players of the team other then the best player be x.
Actual average = \frac{(x+50)}{5}
Required average = 75 = \frac{(x+80)}{5}
x = (75\times 5)-80
x = 375-80
x = 295
Total points scored by the team = 295 + 50 = 345
Type 3: How to solve Problems on Average speed distance time
Question 1 : Tushar travels from his home to office at the speed of 20 Kmph. While returning from office to home his speed increases by 20%. Calculate Tushar’s average speed.
Solution : Tushar’s speed while returning home = 120\times \frac{20}{100} = 24Kmph
Average speed = \frac{(2ab)}{a+b}
That is, \frac{(2\times 20\times 24)}{(20+24)} = \frac{960}{44} = 21.81Kmph
Therefore, the average speed of Tushar will be 21.81 Kmph.
Question 2 : Shyam travels at speed 40 km/hr for half the time and speed 30 km/hr for other half of the time. Then,what will be average speed of Shyam?
Solutions : Average speed of Shyam = \frac{a+b}{2} = \frac{40+30}{2} = 35
Question 3 : Ravi traveled a distance of 500 km partially on a motorbike and partially in car. The speed of the motorbike was 50 Kmph and distance covered was 200 Km. The total time taken in the journey was 6 hours. Find the average speed for the entire journey.
Solution : Average Speed = \frac{\text{Total Distance}}{\text{Total Time}}
Average speed = \frac{500}{6}
= 83.33 Kmph
Type 4: How to solve Average Problems on Numbers
Question 1: The average of 5 numbers is 50. The average of the first and the second number is 40. Similarly, the average of the fourth and the fifth number is 25. Find the third term of the series.
Solution: Let the third term be x
Average of 5 numbers =50
Therefore, the sum total of all 5 numbers = 50 x 5 = 250
Sum of first two numbers = 40 x 2 = 80
Sum of fourth and fifth term = 25 x 2 = 50
80+x+50 = 250
x = 250-130
x = 120
Question 2 : The average of 5 consecutive numbers is 50. Find the numbers.
Solution: Let the numbers be (n-2), (n-1), (n), (n+1), (n+2)
Sum of all numbers = n-2+n-1+n+n+1+n+2 = 50 x 5
5n = 250
n = 50
Numbers = 48, 49 , 50, 51, 52
Question 3 : Calculate the average of 5 consecutive odd numbers greater than 15.
Solution: Odd numbers greater than 15 = 17, 19, 21, 23, 25
Average = \frac{(17+19+21+23+25)}{5} = 21
Alternatively:
There are 5 terms which are at equal interval so, 21 will be Average
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Allegations and Mixtures – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Ratio and Proportions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Simple & Compound Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Simple Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Compound Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Percentages – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Profit & Loss – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
Others
- Allegations and Mixtures – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Ratio and Proportions – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Simple & Compound Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Simple Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Compound Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Percentages – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Profit & Loss – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts
Others

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Login/Signup to comment