Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
Geometric Progression Questions and Answers
Geometric progression Questions
In this page Geometric Progression Questions and Answers is given for Your Practice . This will help you in Solving Questions of Geometric Progression.
Definition of GP
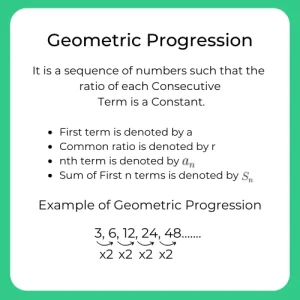
A Geometric progression is a kind of order that includes an organized and immeasurable assortment of real numbers, wherein every term is acquired by multiplying its previous term through a constant value.

Geometric Progression Questions for Practice
General form of Geometric Progression:
a, ar, ar², ar³, ……..
where,
The first term is denoted as = a
The common ratio is denoted as = r
Types of Geometric Progression
Geometric progression can be classified based on the number of terms in the sequence.
- Finite Geometric Progression: A finite geometric progression is a sequence of numbers that has a fixed number of terms. The general form of a finite geometric progression is:
a, ar, ar^2, ar^3, ar^4, …, ar^(n-1)
For example: 2, 4, 8,16
- Infinite Geometric Progression: An infinite geometric progression is a sequence of numbers that goes on forever. The general form of an infinite geometric progression is :
a, ar, ar^2, ar^3, ar^4, …,
For example: 2, 4, 8, …………
Application of Geometric Progression is in physics, engineering, biology, economics, computer science, queueing theory, and finance that’s why Geometric Progression questions and answers are important to know.
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Also Check Out

×
Please login to report
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Linear Equations – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Quadratic Equations – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Linear Equations –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Quadratic Equations –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates



