How To Solve Inverse Questions Quickly

August 3, 2023

How to Solve Inverse Question Quickly in Aptitude

Here , In this Page you will find, How to Solve Inverse Questions Quickly. Inverse functions has Many importance in Aptitude. It is also used in Geometry.

For a function to have an inverse, the function has to be 1 to 1.  That means every output only has one input. In Algebraic Questions Algebra is involved whereas In Geometric Questions Trigonometry is involved.

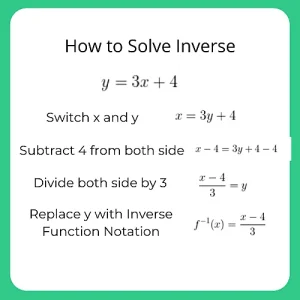
How to Solve Inverse Questions Quickly

How to solve Inverse function

Inverse Rational Function

A rational function is a function of form f(x) = P(x)/Q(x) where Q(x) ≠ 0. To find the inverse of a rational function, follow the following steps. 

  • Step 1: Replace f(x) = y
  • Step 2: Interchange x and y
  • Step 3: Solve for y in terms of x
  • Step 4: Replace y with f-1(x) and the inverse of the function is obtained.

Inverse Questions and Answers

Question 1 : Find an equation for the inverse of the function given f(x) = 3x + 2

Options

A. f-1(x) = \frac{1}{3} \ (x-2)

B.  f-1(x) = \frac{1}{2} \ (x-2)

C. f-1(x) = \frac{3}{2} \ (3x-2)

D,  f-1(x) = \frac{1}{2} \ (2x-1)

Explanations :

First we drop the function notation and write y instead of f(x). Then we solve for x and finally, swap x and y.

y = 3x+2

swap  x and y

x = 3y+2
3y = x-2  (divide by 3)
\frac{x-2}{3} \ = y
f-1(x) = \frac{1}{3} \ (x-2)

Correct Options (A)

Question 2 : Find the inverse of f(x) = \frac{x+4}{3x – 5}

Options

A.  f-1(x) = \frac{5x+4}{3x-1} \

B.  f-1(x) = \frac{5x+3}{3x-1} \

C.  f-1(x) = \frac{2x+3}{5x-1} \

D. None of these

Explanations :

First we drop the function notation and write y instead of f(x). Then swap x and y.

y = \frac{x+4}{3x-5}

x = \frac{y+4}{3y-5}   (multiply by 3y-5)
x(3y-5) = y+4   (distribute)
3xy – 5x = y+4   (add 5x, subtract y)
3xy – y = 5x +4  (factor out y)
y(3x – 1) = 5x + 4  (divide by 3x – 1).

y = \frac{5x+4}{3x-1} \

f-1 (x)= \frac{5x+4}{3x-1} \

Correct option (A)

Question 3 : Find the inverse of f(x) = log _5 (2x-1)

Options

A . f^{-1} = \frac{1}{2} (5^x + 1)

B. f^{-1} = \frac{2}{5} (2^x + 1)

C. f^{-1} =  \frac{3}{5} (x+ 1)

D. None of these

Explanations :

y = log _5 (2x-1)   (re-write it as exponential statement.

5^{y} = 2x – 1

Swap x and y

5^{x} = 2y – 1(add 1)
5^{x} + 1 = 2y (divide by 2)
\frac{5^x + 1}{2} \ = y
y = \frac{1}{2}(5^x + 1)
f-1 = \frac{1}{2} (5^x + 1)

Correct Options (A)

Question 4 : What  is the inverse function of tan(x)?

Options

A. cos^{-1}(x)

B. tan^{-1}(x)

C. tan^{-1}(\frac{1}{x})

D. None

Solution :

y = tan(x)

x = tan(y)

y = tan^{-1}(x)

Correct Option B

Question 5:  What is the value of sin(sin^{-1}(x)) where x belongs to [-1,1]

Options

A. 1

B. 0

C. x

D. None

Solution:

In this Expression sin(sin^{-1}(x))

Domain of sin^{-1}(x) is [-1,1] so it’s value will be x

Prime Course Trailer

Related Banners

Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription

Get Prime

Also Check Out

Questions and Answers to solve Inverse
Formulas to solve Inverse Questions quickly
Tips, Tricks and shortcuts for Inverse
Branding

Get over 200+ course One Subscription

Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others

Get Prime
Course List
Course List

Checkout list of all the video courses in PrepInsta Prime Subscription

Checkout list of all the video courses in PrepInsta Prime Subscription

Checkout
Checkout